1995

Tests of QED in High-Z One- and Few-Electron Systems

Ground State Lamb Shift of H-like Uranium

One of the most sensitive experimental approaches for the investigation of the effects of quantum electrodynamics in strong Coulomb fields is a precise determination of the ground state energies in high-Z one-electron systems. Similar investigations for low-Z ions are primarily sensitive to the lowest-order self-energy corrections. The study of the higher-order self-energy and vacuum polarization contributions, however, requires the heaviest ions available [1]. Here, the goal of the experiments is to probe higher-order QED contributions which correspond to Feynman diagrams such as the two-photon exchange diagrams. For the case of uranium, where the total 1s Lamb shift contributes 464 eV to the total ground state binding energy of 131.816 keV, a stringent test of QED requires an absolute experimental accuracy of about ± 1 eV which represents the accuracy theoreticans claim presently. For such studies the ESR storage ring provides favorable experimental conditions. This has been demonstrated within the first series of experiments performed at the ESR gas jet target [2] as well as at the electron cooler device [3]. For the case of the 1s-Lamb shift in hydrogen-like uranium, the achieved accuracy of ± 16 eV is already a substantial improvement by almost one order of magnitude compared to a former experiment conducted at the BEVALAC accelerator [4]. However, the available results are still at the threshold of a real test of higher-order QED contributions.

X-Ray Spectra of H-like Uranium

 

 

 

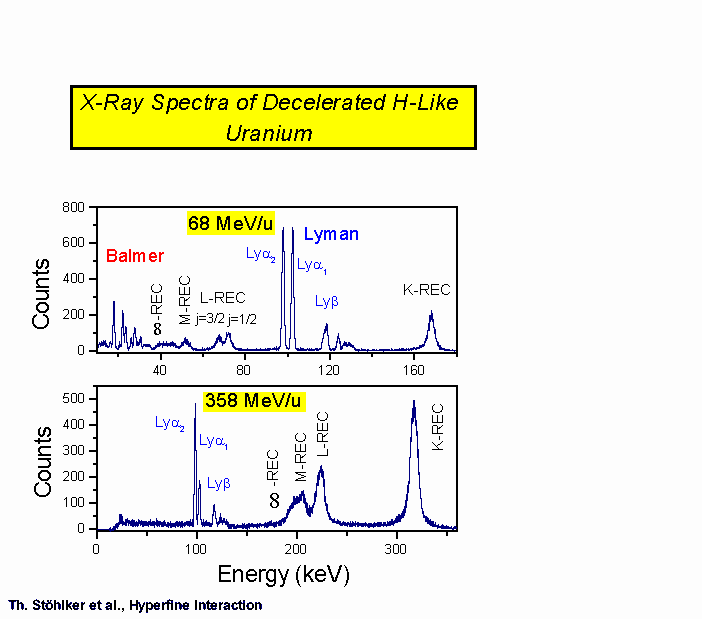
Figure 1: X-ray spectrum (projectile system) of H-like uranium measured at 68 MeV/u (top) in comparison with the one recorded at 358 MeV/u (bottom).

In the second part of the experiment the deceleration option was applied. After finishing the stacking procedure, which was still performed at the energy of 360 MeV/u, the coasting DC-beam was rebunched and decelerated by simultaneously ramping down the magnetic fields. Subsequently, electron cooling was switched on in order to balance the beam energy loss in the gas target and to fix the ion velocity to the chosen final beam energies of 220, 68, and 49 MeV/u. By applying this procedure up to 2* 10⁷ ions could be decelerated with losses below 20%. At the two lowest beam energies, the electron cooler current was kept on a comparably low value of 50 mA in order to avoid a too strong reduction of the beam lifetime. It is important to note that, still at the lowest energy, charge-exchange in the residual gas has not caused any significant beam losses. For the experiment with the decelerated ions a N₂ gas-jet target with a low areal density of about 10¹¹ particles/cm² was applied. The large reaction cross-sections of the gaseous target reduced drastically the beam lifetime to 5 min at 68 MeV/u and to about 1 min at 49 MeV/u. The potential of the deceleration capabilities of the ESR is illustrated by the spectra of hydrogen-like uranium shown in Fig. 1. Compared to the high beam energy of 358 MeV/u, the x-ray spectra recorded for the decelerated ions (68 MeV/u) provide an abundant yield of different characteristic projectile transitions. At the high-energy the spectrum is almost entirely governed by Radiative Electron Capture (REC) transitions into the ground and excited states of the projectile. In contrast, the spectrum recorded for decelerated uranium is dominated by the characteristic Lyman transitions (Lya ₁: 2p_3/2=>1s_1/2; Lya ₂: 2p_1/2 =>1s_1/2., where the latter is blended by the 2s_1/2 =>1s_1/2 M1 transition). These transitions give the most direct access for the study of the ground state QED properties.

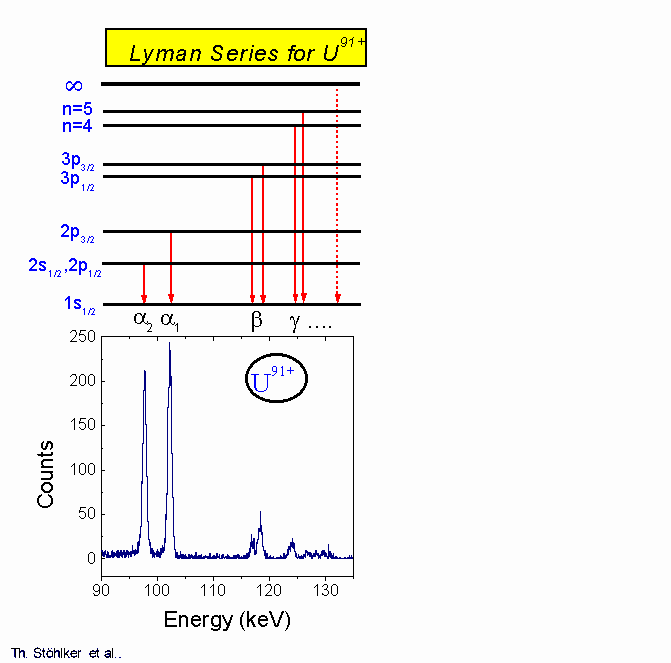
Figure 2: Lyman spectrum of H-like uranium (projectile system) measured by one segment of a strip detector at 48 deg observation angle.

In Fig. 2 the transformed Lyman spectrum (projectile system) is shown recorded by one segment of a strip detector mounted at 48 deg (68 MeV/u, U⁹²⁺ =>N₂ collisions). The most striking feature of this spectrum is the observed splitting of the Lyman-b line into two components (3p_1/2 =>1s_1/2 and 3p_3/2  =>1s_1/2 transitions) which makes this experiment also sensitive to the M-shell fine structure splitting. This gain in resolution is a consequence of the strongly reduced Doppler broadening  dE_LAB/E_LAB = 5* 10^-3 caused by the small angle acceptance of ± 0.32 deg of this particular detector as well as by the low ion velocity. Complementary information on the Lyman spectrum is provided by the Balmer transitions. In Fig. 3 the Balmer spectrum (projectile system) is plotted as it was measured by a conventional Ge(i) detector installed at an observation angle of 48 deg. In addition, a level scheme is shown illustrating the origin of the most prominent transition lines. The various transitions between the M- and L-subshell levels are clearly distinguished, and transitions from higher states up to the series limits can be identified as well. Note that these transitions are almost uneffected by QED corrections, and therefore may serve as an intrinsic Doppler correction for the measured x-ray spectra.
In conclusion, a dedicated ground state QED experiment has been performed for H-like uranium by utilizing for the first time the deceleration mode of the ESR. The x-ray spectra recorded provide an abundant yield of different characteristic projectile transitions. They illustrate the considerable progress made for spectroscopic experiments dealing with high-Z ions. Compared to former experiments performed at the gas target one expects from the applied deceleration technique a significant improvement in the absolute accuracy of the final results. Moreover, within the near future, a further progress towards an absolute accuracy of ± 1 eV may be anticipated. The high-intensity beams of about 10⁸ bare uranium ions, already available from the SIS, along with a strongly enhanced injection efficiency into the ESR, will allow the implementation of high-resolution x-ray detection devices such as crystal spectrometers or bolometers which presently are under construction.  
 

Figure 3: Balmer spectrum of H-like uranium (projectile system) measured by a Ge(i) x-ray detector at 48 deg observation angle

 

 

Ground State Investigations for High-Z, He-like Ions

Besides the one-electron systems, the two-electron ions are of particular interest for atomic structure studies as they represent the simplest multi-electron systems. Investigations of these ions along the isoelectronic sequence probe uniquely our understanding of correlation, relativistic and quantum electrodynamical effects. Very recently the theoretical and the experimental investigations of these fundamental systems achieved a considerable improvement in accuracy. In theory a new generation of relativistic many-body calculations has established significantly improved benchmarks for the non-QED part in the electron-electron interactions which are now considered within all orders. For the ground state the progress which has taken place during the last year is particularly impressive, since even the two-electron QED effects can presently be calculated complete to second order, i.e. all the two-photon contributions to the electron-electron interaction are included in the newest calculations [6]. Experimentally, the two-electron contributions to the ground state energy of He-like ions can now be studied uniquely at storage rings and traps by measuring the Radiative Recombination (RR) transitions into the ground state of H-like ions relative to the one into the bare species [7]. Since RR is the time reversal of the photoelectric effect, the difference between both RR transitions energies is equal to the difference in the ionization potentials between the one- and the two-electron system. In particular, all one-electron contributions to the binding energy cancel out completely in this type of experiment. This novel technique has been introduced for the very first time in an experiment conducted at the Super Electron Beam Ion Trap (SEBIT) at the Lawrence Livermore National Laboratory [7]. In the experiment results were obtained for various elements with nuclear charges between 32 and 83. In Table 1 the experimental results are given in comparison with the individual two-electron contributions as predicted by Persson et al. [6]. Although the experimental accuracy is only at the threshold of testing the predicted two-electron QED contributions, the measurements provide a meaningful test of the many-body part of the theory. In particular, an improvement of only half an order of magnitude is required to test the two-electron QED effects which are caused by the exchange of two virtual photons. The ESR seems to be particularly well suited for such future investigations. Due to its capability to store simultaneously highly-charged heavy ions with the same atomic number Z but with different charge states, such a relative measurement can be conducted at the cooler section of the storage ring.

Table 1: Absolute size (in eV) of the individual two-electron contributions to the ground state binding energy in some He-like ions [6] in comparison with the experimental results from Super-EBIT [7] (AS: non-radiative QED, the Araki Sucher term; VP: two-electron vacuum polarization; SE: two-electron self energy; Total theory: predicted difference in the ionization potentials between the H and the He-like system).

Z	non-QED part	AS	VP	SE	Total theory	Experiment
32	562.41	0.0	0.0	-0.5	562.0	562.5 ± 1.6
54	1029.55	0.2	0.2	-1.8	1028.2	1027.2 ± 3.5
66	1338.89	0.4	0.6	-3.2	1336.6	1341.6 ± 4.3
74	1577.06	0.6	0.9	-4.6	1573.9	1568 ± 15
83	1885.83	0.9	1.6	-6.7	1881.5	1876 ± 14

 

2s - 2p Transitions in Helium- and Lithium-like Ions

In contrast to the ground state of He-like ions, the predictions for the QED contributions to the excited L-shell levels in He- and Li-like ions are still incomplete whereas the many-body non-QED contributions can now be calculated accurately. Experimentally, the 2s - 2p transitions are most appropriate for a test of atomic structure calculations as these transitions are comparatively strongly influenced by QED effects. However, the available numbers of precise measurements with sensitivity to the two-photon QED contributions is very limited. In particular there is a lack of data in the high-Z regime. For Z > 36 there are no precise He-like 2s-2p measurements, and for Li-like ions the most precise 2s-2p measurements have been reported for Z=42 from a tokamak [8], for Z=90 and 92 from SEBIT [9] and for Z=92 using Doppler-tuned spectroscopy [10]. All the high-Z 2s-2p transition energy measurements show reasonable agreement with theory. The Doppler-tuned spectroscopy measurement of the 2s ²S_1/2 -2p²P_1/2 transition in Li-like U⁸⁹⁺ is sensitive to QED contributions to within 0.3%. This is similar to the best reported QED sensitivity in He-like ions for the 2s ³S₁ -2p³P₀,₂ transitions from a beam-foil measurement in Ar¹⁶⁺[11]. At GSI a precise beam-foil measurement of the 2s² S_1/2 -2p²P_3/2 transition in Li-like Ni was performed by using the GSI/Giessen VUV spectrometer at the UNILAC [12]. The measurement demonstrates the capability of the spectrometer and allows, in comparison with the data from tokamaks, a critical test of the new experimental installations which are developed for future experiments dealing with heavier He- and Li-like ions. A wavelength of l =16.5382 nm ± 0.0024 nm (74.968 eV ± 0.0109 eV) was measured which corresponds to a relative precision in the determination of the transition energy of 1.5* 10^-4. In particular, a comparison with the results obtained at tokamaks shows a very good agreement. In table 2 the size of the various theoretical QED contributions to the 2s² S_1/2 -2p²P_3/2 transition energy in Ni²⁵⁺ [13] is compared with the experimental results. The measured QED value was established by subtracting the calculated non-QED part from the measured transition energy. The achieved experimental accuracy provides a test of the QED contributions to within 1.9% and confirms the significance of the two-photon QED contributions (see table 2). Note that an extrapolation of this relative accuracy to a measurement of the 2s²S_1/2 -2p²P_1/2 transition energy in Li-like Sn⁴⁷⁺ would test the QED contributions to within 0.3%.

Table 2: The individual theoretical effects (in eV) contributing to the 2s²S_1/2 -2p²P_3/2 transition energy for Li-like Ni in comparison with the experimental QED result [12] (the theoretical predictions were gained from Ref. [9] by a cubic spline interpolation). Here SE denotes the one-valence-electron self energy; VP the one-valence-electron vacuum polarization, X and C the two-photon QED contributions of the valence state and core state, respectively; QED_Calc the predicted total QED contribution; QED_Exp the measured QED result. The given error denotes the total experimental uncertainty.

SE	VP	X	C	QEDCalc	QEDExp
-0.6442	0.0557	0.0122	-0.0081	-0.5844	-0.5800 ± 0.0109

 

Literature:

[1] W.R. Johnson and G. Soff, At. Data and Nucl. Data Tables 33, 405 (1985).
[2] Th. Stöhlker et al. Phys. Rev. Lett. 71, 2184 (1993).
[3] H.F. Beyer et al., Phys. Lett  A814, 435 (1994);
     H.F. Beyer et al., Z. Phys. D35, 169 (1995).
[4] J.P. Briand et al., Phys. Rev. Lett 65, 2761 (1990).
[5] Th. Stöhlker, GSI-Nachrichten 05-95 (1995).
[6] H. Persson, et al. Phys. Rev. Lett. 76, 204 (1996).
[7] R.E. Marrs et al., Phys. Rev. A52,3577 (1995)
[8] E. Hinnov and B. Denne, Phys. Rev. A40, 4357 (1989);
     J.  Sugar et al., Opt. Soc. Am. B9, 344 (1992).
[9] P. Beiersdorfer et al., Phys. Rev. Lett 71, 3939 (1993);
     P. Beiersdorfer et al., Phys. Rev. A52, 2693 (1995).
[10] J. Schweppe et al., Phys. Rev. Lett. 66, 1434 (1991).
[11] K. Kukla et al., Phys. Rev. A51, 1905 (1995).
[12] R. Büttner et al., Nucl. Instr. Meth. B98, 41 (1995).
[13] S.A. Blundell, Phys. Rev. A47, 1790 (1997)

 

X-Ray Emission from High-Z Projectiles Colliding with Gaseous Matter

Th. Stöhlker, T. Ludziejewski, F. Bosch, C. Kozhuharow, P.H. Mokler, H. T. Prinz, H. Reich, H.F. Beyer, G. L. Borchert, R. W. Dunford, J. Eichler, B. Franzke, A. Gallus, H. Geissel, H. Gorke, A. Ischihara, D. Ionescu, A. Kräamer, D. Liesen, A. E. Livingston, G. Menzel, P. Rymuza, C. Scheidenberger, T. Shirai, Z. Stachura, L. Stennar, M. Steck, P. Swiat, T. Winkler, and A. Warczak

(PS-FILE: 9029 KB)